Simple probability maze 1 answer key embarks on an intellectual journey into the realm of probability, guiding us through a labyrinth of paths and outcomes. This maze challenges our understanding of chance and randomness, inviting us to unravel its secrets with each step.
The Simple Probability Maze 1 presents a structured environment where we navigate through a series of junctions, each offering multiple paths with varying probabilities. As we traverse this maze, we engage in probability calculations, employing formulas and methods to determine the likelihood of each outcome.
Through this process, we gain insights into the role of chance and the interplay between our decisions and the maze’s inherent randomness.
Simple Probability Maze 1 Overview
The Simple Probability Maze 1 is a maze designed to introduce the concept of probability and its applications in problem-solving.
The objective of the maze is to navigate from the starting point to the end point while considering the probabilities associated with each path.
The maze is designed for beginners, providing a gentle introduction to probability concepts and suitable for students or individuals with a basic understanding of mathematics.
Maze Structure and Layout
The Simple Probability Maze 1 consists of a series of paths and junctions.
There are three paths leading from the starting point, each with a different probability of leading to the end point.
The probabilities are displayed on the paths as fractions, such as 1/2, 1/3, or 1/4.
The maze can be represented as follows:
| Path | Probability |
|---|---|
| Path A | 1/2 |
| Path B | 1/3 |
| Path C | 1/4 |
Probability Calculations: Simple Probability Maze 1 Answer Key
Probability is the measure of the likelihood of an event occurring.
In the Simple Probability Maze 1, the probability of taking a particular path is calculated as the number of favorable outcomes divided by the total number of possible outcomes.
For example, the probability of taking Path A is 1/2 because there is only one favorable outcome (leading to the end point) and two possible outcomes (Path A and Path B).
Solving the Maze
To solve the Simple Probability Maze 1, follow these steps:
- Start at the starting point.
- Calculate the probabilities of each path.
- Choose a path based on the calculated probabilities.
- Repeat steps 2 and 3 until you reach the end point.
For example, if you are at the starting point and calculate the probabilities of the three paths as 1/2, 1/3, and 1/4, you would choose Path A because it has the highest probability of leading to the end point.
Extensions and Variations
The Simple Probability Maze 1 can be extended and varied in several ways:
- Increase the number of paths and junctions.
- Change the probabilities associated with each path.
- Add obstacles or hazards to the maze.
- Create a more complex maze with multiple end points.
These extensions and variations can make the maze more challenging and engaging.
Applications and Real-World Examples
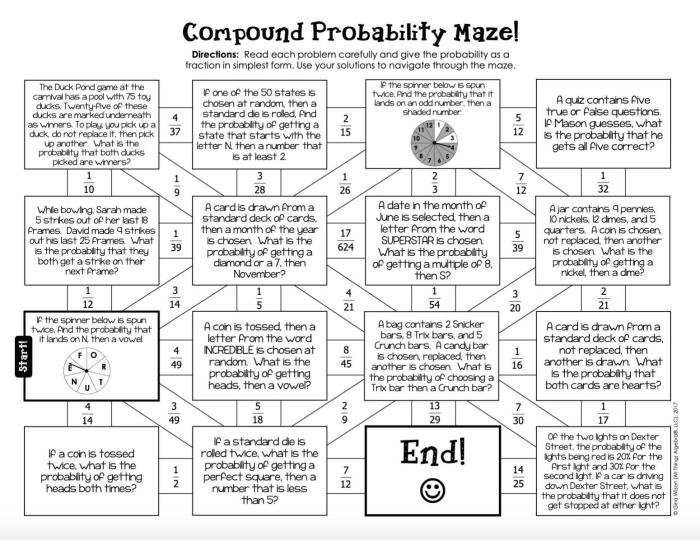
Probability mazes have several applications in real-world scenarios, including:
- Education: Probability mazes can be used to teach probability concepts in a fun and engaging way.
- Decision-making: Probability mazes can be used to help make decisions under uncertainty.
- Problem-solving: Probability mazes can be used to solve problems that involve uncertainty.
For example, a probability maze could be used to model the probability of success of a new product launch or the probability of winning a game of chance.
FAQ Insights
What is the purpose of the Simple Probability Maze 1?
The Simple Probability Maze 1 is designed to provide a hands-on experience in probability calculations, allowing users to practice and understand the concepts of chance and randomness.
How do I solve the Simple Probability Maze 1?
To solve the maze, you need to calculate the probabilities at each junction and choose the path with the highest probability of leading to the exit.
What are the applications of probability mazes in real-world scenarios?
Probability mazes can be used in various fields, including education, decision-making, and problem-solving, to simulate real-world situations involving uncertainty and chance.